The thermodynamic cycles, in general, may be classified into the following two types;
1. Reversible Cycle or ideal cycle
2. Irreversible or natural or real cycle
1. Reversible Cycle or ideal cycle
2. Irreversible or natural or real cycle
 An isolated system is more restrictive than a closed system as it does not interact with its surroundings in any way. Mass and energy remains constant within the system, and no energy or mass transfer takes place across the boundary. As time passes in an isolated system, internal differences in the system tend to even out and pressures and temperatures tend to equalize, as do density differences. A system in which all equalizing processes have gone practically to completion is considered to be in a state of thermodynamic equilibrium.
An isolated system is more restrictive than a closed system as it does not interact with its surroundings in any way. Mass and energy remains constant within the system, and no energy or mass transfer takes place across the boundary. As time passes in an isolated system, internal differences in the system tend to even out and pressures and temperatures tend to equalize, as do density differences. A system in which all equalizing processes have gone practically to completion is considered to be in a state of thermodynamic equilibrium. ) have a specific value corresponding to that state. The values of these properties are a function of the state of the system. The number of properties that must be specified to describe the state of a given system (the number of degree of freedom) is given by Gibbs phase rule:
) have a specific value corresponding to that state. The values of these properties are a function of the state of the system. The number of properties that must be specified to describe the state of a given system (the number of degree of freedom) is given by Gibbs phase rule: 3N/2(2mE)3N/2VN]/[(N!
3N/2(2mE)3N/2VN]/[(N! (3N/2)]}(
(3N/2)]}( E/E), where
E/E), where p = (2mE)1/2(
p = (2mE)1/2( E/2E) is the range of momentum,
E/2E) is the range of momentum, 3N/2(2mE)(3N-1)/2 comes from integrating up to the energy E = p2/2m,
3N/2(2mE)(3N-1)/2 comes from integrating up to the energy E = p2/2m, (3N/2) are for removing the degeneracy related to the permutation symmetry of identical particles.
(3N/2) are for removing the degeneracy related to the permutation symmetry of identical particles.  (3N/2) is the Gamma function identical to (3N/2)! if the argument is an integer.
(3N/2) is the Gamma function identical to (3N/2)! if the argument is an integer. E of the phase space. The Planck's constant h = 6.625x10-27 erg-sec from the uncertainty relation
E of the phase space. The Planck's constant h = 6.625x10-27 erg-sec from the uncertainty relation  p
p x ~ h in quantum theory is conveniently taken as the basic unit (minimum size) of the microscopic states. Thus the partition function Z is just:
x ~ h in quantum theory is conveniently taken as the basic unit (minimum size) of the microscopic states. Thus the partition function Z is just: 1/2(2mE)1/2V1/3)/h]3N/[N!
1/2(2mE)1/2V1/3)/h]3N/[N! (3N/2)]}(
(3N/2)]}( E/E) ~ {(108)3N/[N!
E/E) ~ {(108)3N/[N! (3N/2)]}(
(3N/2)]}( E/E)
E/E) dN ---------- (2)
dN ---------- (2) is the chemical potential.
is the chemical potential. ) is defined as mass per unit volume.
) is defined as mass per unit volume. ) of a thermodynamic system is the change in the energy of the system when a different kind of constituent particle is introduced, with the entropy and volume held fixed.
) of a thermodynamic system is the change in the energy of the system when a different kind of constituent particle is introduced, with the entropy and volume held fixed.

 In open systems, matter may flow in and out of the system boundaries. The first law of thermodynamics for open systems states: the increase in the internal energy of a system is equal to the amount of energy added to the system by matter flowing in and by heating, minus the amount lost by matter flowing out and in the form of work done by the system. The first law for open systems is given by:
In open systems, matter may flow in and out of the system boundaries. The first law of thermodynamics for open systems states: the increase in the internal energy of a system is equal to the amount of energy added to the system by matter flowing in and by heating, minus the amount lost by matter flowing out and in the form of work done by the system. The first law for open systems is given by:



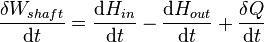
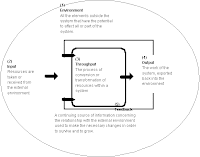
 All space in the universe outside the thermodynamic system is known as the surroundings, theenvironment, or a reservoir. A system is separated from its surroundings by a boundary which may be notional or real, but which by convention delimits a finite volume. Exchanges of work, heat, or matter between the system and the surroundings may take place across this boundary. Thermodynamic systems are often classified by specifying the nature of the exchanges that are allowed to occur across its boundary.
All space in the universe outside the thermodynamic system is known as the surroundings, theenvironment, or a reservoir. A system is separated from its surroundings by a boundary which may be notional or real, but which by convention delimits a finite volume. Exchanges of work, heat, or matter between the system and the surroundings may take place across this boundary. Thermodynamic systems are often classified by specifying the nature of the exchanges that are allowed to occur across its boundary. Thermal Mass Flow (TMF) technology uses thermodynamic principles to drive actual mass flow. A thermal mass flow sensor can be combined with an integral valve and PID flow controller in one compact, efficient instrument that can accurately control the flow of gases and liquids over a wide range of flow rates.
Thermal Mass Flow (TMF) technology uses thermodynamic principles to drive actual mass flow. A thermal mass flow sensor can be combined with an integral valve and PID flow controller in one compact, efficient instrument that can accurately control the flow of gases and liquids over a wide range of flow rates. Aluminium fabrication is a challenging task indeed. The special properties of aluminium often pose some serious challenges even to the most experienced welders. For example, aluminum is a very well conductor of heat. At the same time, it has a low melting point. Naturally, it becomes difficult to weld the aluminium sheet without burning at least some portions of it. Also, there are some impurities involved in the aluminium which is important to be got rid of. At the same time, one should preheat the aluminium sheet to ensure that the welding is done properly without any chance of cracks.
Aluminium fabrication is a challenging task indeed. The special properties of aluminium often pose some serious challenges even to the most experienced welders. For example, aluminum is a very well conductor of heat. At the same time, it has a low melting point. Naturally, it becomes difficult to weld the aluminium sheet without burning at least some portions of it. Also, there are some impurities involved in the aluminium which is important to be got rid of. At the same time, one should preheat the aluminium sheet to ensure that the welding is done properly without any chance of cracks.